The latest issue of Earth-Science Reviews contains a couple of articles where the issue of scale linkage is front and center. Ma et al. (2017) review the past five years or so of research on hydropedology, focusing on soil-water interactions across spatiotemporal scales. Walker et al. (2017) outline scale-dependent perspectives on geomorphic evolution of beach and dune systems, based largely on years of collaborative work on Prince Edward Island (Canada).

Beach and frontal dune at Prince Edward Island National Park (http://www.parkscanada.gc.ca/pei)
Both papers deal with a classic, hoary problem in the geosciences—but a problem that still has not been fully solved, and that vexes researchers regularly. Soil, hydrologic, geomorphic, ecological, and climate systems are all intertwined, and all are influenced by processes ranging from molecular to planetary, and operating over time scales from instantaneous to eons. In some senses these processes and controls as they apply to a given Earth surface system (ESS) are connected and related. However, in practical terms the details of geochemical kinetics or wind stress on a sand grain do not apply to the geological evolution of landscapes; nor do paleogeography, plate tectonics, and evolution of the atmosphere explain the response of a soil or sand dune to a storm. At the far end of the ranges, intuition and common sense alone is sufficient to justify some exclusions. For example, no one expects Walker and company to address geological evolution of the Canadian continental margin in their geomorphological studies of Prince Edward Island; no one would demand that Ma et al. place their work in a palaeopedological context. Likewise, in my study of fluviokarst landscape evolution, no reviewer insisted that I address the partial pressures of dissolved carbon dioxide. Of course, there’s a lot of room between the extremes of geological time and the time spans of fluid flows and chemical reactions.
More than half a century ago Schumm and Lichty (1965) produced a classic statement pointing out that whether variables are dependent or independent depends on temporal scale, using examples from geomorphology. The intuitive truth of this can be formally demonstrated. For example, for an Earth surface system characterized as a set of interacting components that operate at fundamentally different scales, it has been proved that the dynamics at different scales are independent in terms of their influences on system dynamics (Schaffer, 1981; Phillips 1986; 1988). Walker et al. (2017) embed their work in Schumm and Lichty’s framework, and extend scale considerations by providing a detailed example of “scale-aware” (their term) conceptual and functional integration of plot and landscape scales.

The dynamical stability or instability of weathering systems varies with spatial scale (Figure 6 from Phillips, 2005).
Ma et al. (2017) explicitly promote a hierarchical framework for spatial scale bridging in hydropedology. They maintain that soil physicists and hydrologists have generally viewed the relevant processes as a continuum, though I would argue that an implicit hierarchy has long existed in field-based hydrological studies.
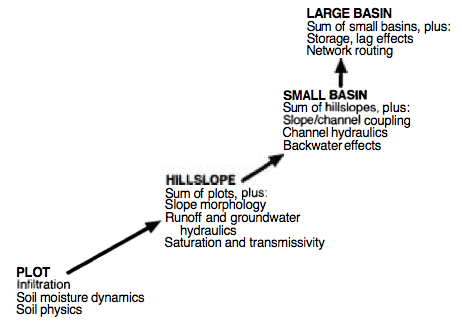
Scale hierarchy in hydrology I proposed in 1999 (Figure 8.1 from Phillips, 1999). Each level includes the cumulative effects of lower levels and new considerations.
The hierarchical approach both Ma et al. (2017) and Walker et al. (2017) promote and illustrate is a sound one, involving linking or bridging between adjacent levels of a scale hierarchy. One formal approach is hierarchy theory, one formal expression of which is:
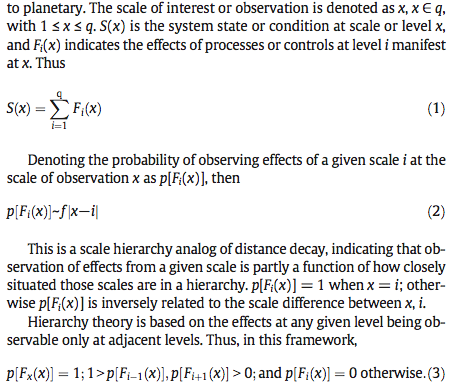
(Excerpt from Phillips, 2016, p. 67).
If the key components of the system are represented as a mathematical graph or network at hierarchical scale levels, it can be shown that as the number of levels increases (that is, consideration is extended up or down the scale hierarchy to broader spatial and longer temporal scales or finer spatial and shorter temporal scales) complexity increases, but at a less than linear rate. However, inferential synchronization (the ability to infer dynamics at one level from those at another) deteriorates rapidly, at a greater than linear rate. This is the phenomenon of scale decay, directly analogous to the geographical concept of distance decay (near things are more closely related than far things). For example, using the algebraic graph theory metrics of spectral radius (and closely related graph entropy) to measure complexity, and algebraic connectivity as a measure of inferential synchronization, we inevitably find something like the trends below. These are for specific examples from pedology (top) and fluviokarst flow patterns (bottom), but similar trends are found in various archetypal hierarchical graph structures.
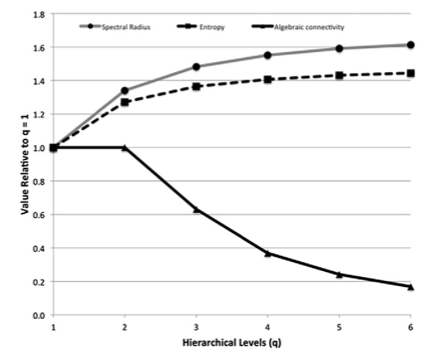
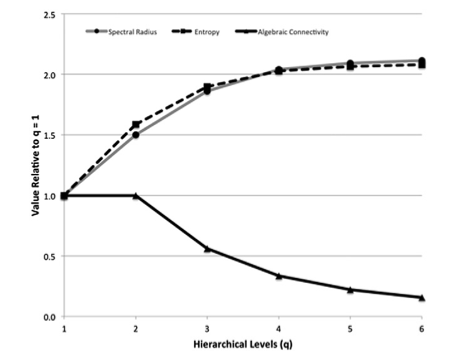
Figures 5 and 7 from Phillips, 2016.
A set of components operating at a given scale can be considered a subgraph or subnetwork of the larger ESS. The eigenvalues (lambda) of the subgraph a are related to those of the larger system by

The unindexed lambdas are the eigenvalues of the larger system, and index d signifies a subgraph consisting of those components not included in a. The eigenvalues (the graph spectrum) describe its dynamics, and this relationship shows that the subgraphs at different scales are independent.
When common sense, intuition, empirical evidence, and formal theory all converge to the same conclusion, I think it is safe to conclude that the conclusion is sound. Scale linkage is important, but a single representation or transfer across a broad range of scales will not work. Rather, a transfer one level at a time up or down a scale hierarchy is the way to go.
References:
Ma Y, and 3 others. 2017. Hydropedology: Interactions between pedologic and hydrologic processes across spatiotemporal scales. Earth-Science Reviews 171: 181-195.
Phillips JD. 1986. Sediment storage, sediment yield, and time scales in landscape denudation studies. Geographical Analysis 18: 161-167.
Phillips JD. 1988. The role of spatial scale in geomorphic systems. Geographical Analysis 20: 359-368.
Phillips, J.D. 1999. Earth Surface Systems. Complexity, Order, and Scale. Oxford, UK: Basil Blackwell.
Phillips JD. 2005. Weathering instability and landscape evolution. Geomorphology 67: 255-272.
Phillips JD. 2016. Vanishing point: scale independence in geomorphic hierarchies. Geomorphology 266: 66-74.
Schaffer WM. 1981. Ecological abstraction: the consequences of reduced dimensionality in ecological models. Ecological Monographs 5: 383–401.
Schumm SA, Lichty RW. 1965. Time, space, and causality in geomorphology. American Journal of Science 263: 110-119.
Walker IJ, and 6 others. 2017. Scale-dependent perspectives on the geomorphology and evolution of beach-dune systems. Earth-Science Reviews 171: 220-253.
Posted 12 August 2017
